Exercise Six: Lines In Space

“Lines have many uses in design. They can be used as the axes of solid forms, to describe planes or volumes, and to delineate.”

In our study of lines, we work with eleven curves and the straight line, which constitute our basic line vocabulary. Like the colors in a color chart, there may be many others in between, but they are similar curves with different proportions.

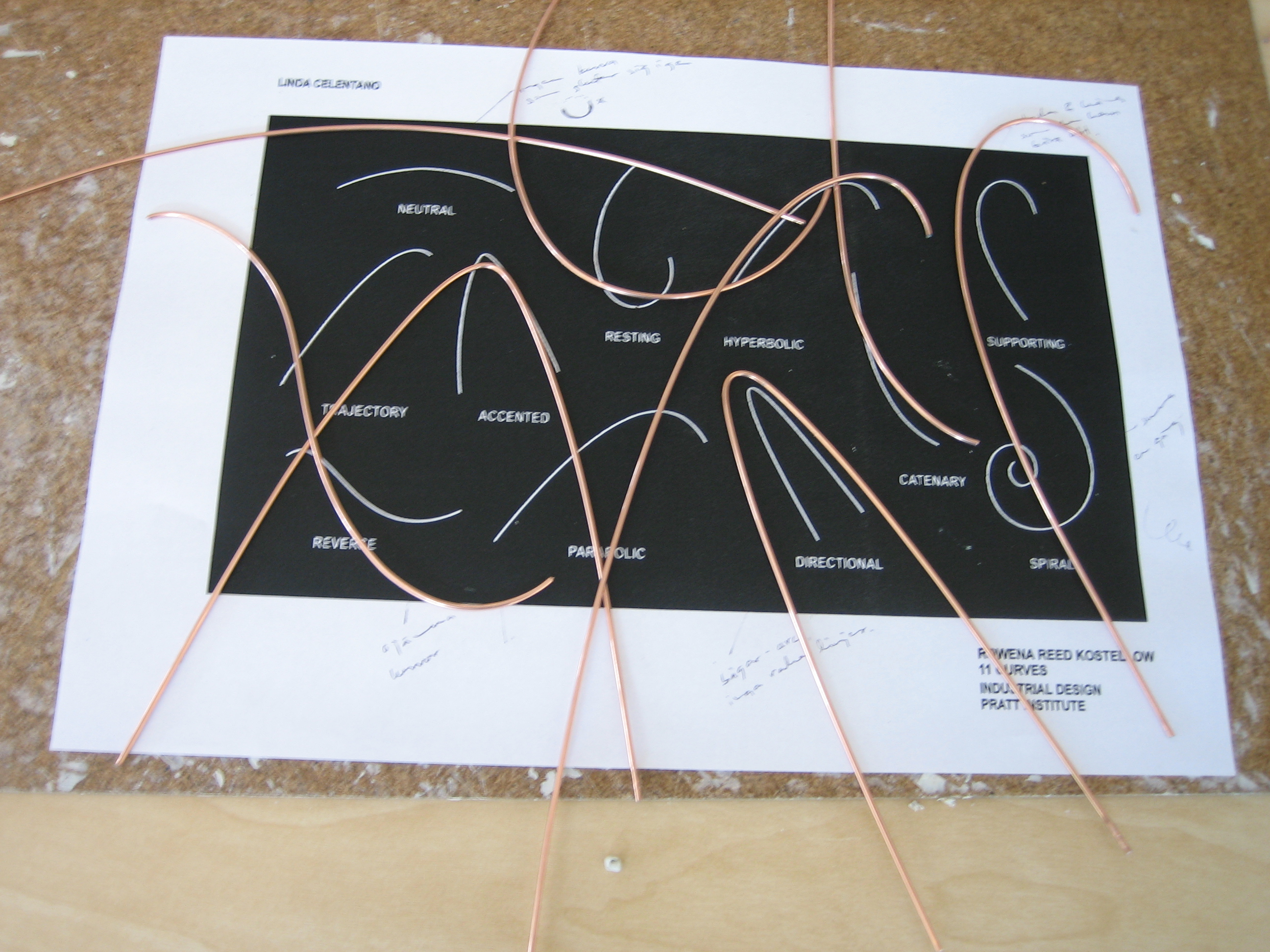
The first three curves are Slower Curves:

  • The neutral curve is the most innocuous and perhaps the least dramatic. It is a segment of a circle. The accent is the same any place you look at it. It expands the same amount throughout its length. (Recall that the accent of a curve is its point of most significant expansion.)
  • The resting curve stands balanced on its accent as if resting lightly on a molecule. It should not flatten out.
  • The supporting curve is just the opposite of the resting curve. If you put something on top of this curve—on the accent—it should feel like it supports the load, like a bridge.

The next four are Faster Curves

  • The trajectory curve is like the path of a ball being thrown or a hose emitting a stream of water. It starts straight and fast, then falls off as the speed diminishes.
  • The hyperbolic curve appears similar to the trajectory but differs in character. It starts straight and fast, but instead of slowly diminishing, it turns back toward the source, concentrating its energy in one spot.
  • The parabolic curve is not the mathematical equivalent of a parabola, but it does resemble one. It is a combination of the trajectory and the hyperbolic: its accent is not as strong as the former or as open as the latter. It is a good curve to use in large-volume, organic forms. When we first began discussing these lines, we called it the General Motors curve because, in those days, all fenders looked like that. It should not be symmetrical—that is, it should not expand equally like a sphere but should have a little accent.
  • The reverse curve is one of the most interesting. It resembles the letter S but should have some verve, motion, and style. It is even more interesting when it has a little diagonal movement.

The next three are Directional Curves

  • The catenary curve is a gravity curve. A chain best demonstrates it. The accent is at its lowest point if you hold one chain end in each hand. Move your hands together; you will get more of an accent; farther apart, you will get less. Moreover, you can move the accent from left to right by lowering one side or the other.
  • The directional curve points like an arrow. One could say that it is not a curve at all but a broken line. It has a powerful directional force.
  • The accented curve is similar to the catenary and directional curves but is slightly curved at the sides, while the others have straight sides.

The last curve is “Independent”

The spiral is hard to combine with other curves. It is a prima donna. It has so many possible accents, depending on the number of spirals within it, that you cannot define them or create tensions between them. If wound loosely, for example, it looks like a snail. If wound very tightly, it is almost like a straight line.

Practice making a variety of curves. To get a feel for these curves, begin by drawing them. Use news sheets and soft charcoal. Work loosely and fast as possible, drawing as many of each in different proportions as possible. When you get the feel of each type, you are ready to make them in wire.

You will need 24 gauge copper wire and needle-nose pliers. The copper wire has an excellent tensional quality, especially after it has been stretched. Pull a length of wire from the spool using the pliers. Hold the end of the wire with the pliers and the wire at the spool with your hand, and pull until the wire is tight. Hold a moment, pull again to stretch the wire, then cut off the stretched piece.

Make your curves in the air; you can see them much better. Mount your best examples on a 24”x36” board. This chart is your alphabet of curves, and you can refer to it when you work on the exercise “Lines in Space.”

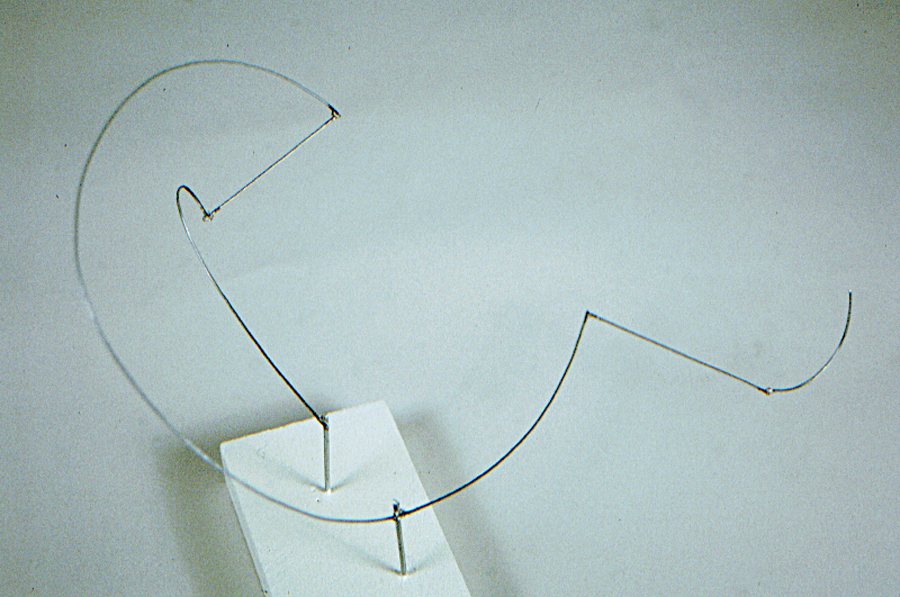
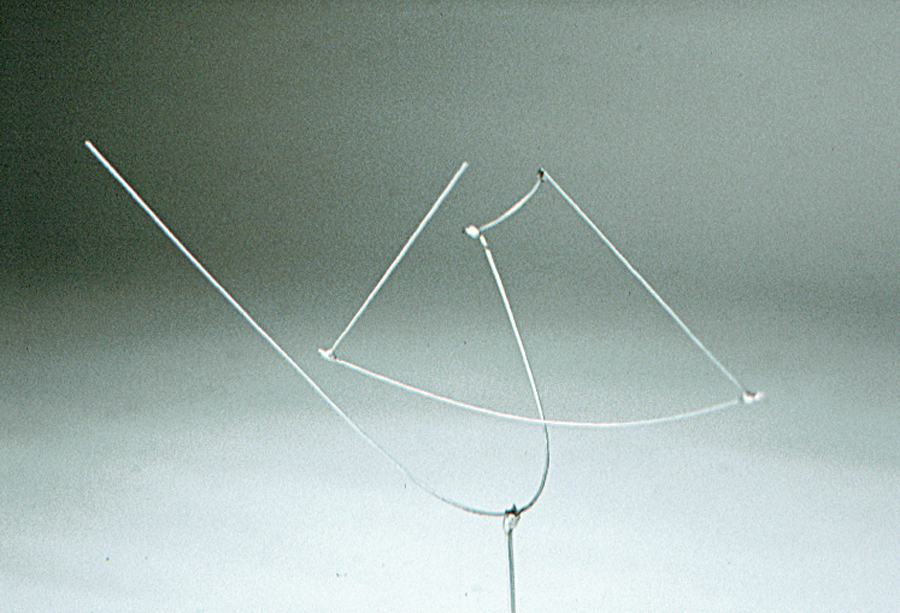
This problem aims to learn how to move lines in and out of space to the maximum. Move in and out as much as possible in the most three-dimensional way. In order to help you do that, I have worked out a formula that forces you to use a variety of curves and some straight lines that contrast with the curves to make the problem more interesting.

Choose four different curves and two straight lines. Of course, many other combinations are possible, but this is a good formula for a first experience. Use contrasting and complementary curves. Divide your lines into two groups of three. You will mount the two groups on a base and connect the two by soldering to form a single design.

Use wood bases (about 1/2” thick), not foam core. You will need the weight of the wood to support the wire. Make a hole with an awl and stick a piece of copper wire into the wood. The wire in your design will readily solder to it, and the connection will be almost invisible. Try to keep all your soldering joints very small.

Start with a piece of stretched wire about 18” long. You are going to make three curves with this piece of wire. Do not start at the bottom and go up. Begin at the top, but do your design about the base. Remember what the bottom will be, and trust your intuitive sense of structure to guide you so that when you mount the wire on the board, it looks like it will stand up. Do not start with a neutral curve because that is not exciting enough.

Design your curve; do not just bend it. Make a curve that uses the tension of the wire. Do not torture the wire too much. Each curve should stay in one plane. As it is, with six curves, you will have six directions.

Decide which position your curve will look best in—where it will have the most character and liveliness. Decide on the plane at right angles to the base or tilted. If you are working with a fast curve, remember that if you keep it at right angles, you will have a fast curve in a static position, which is almost a contradiction.

When you have made your first curve and are ready to make a turn into your next one, hold on to your first curve for dear life, and do not bend your wire by pushing it around. Make a little angle with your pliers, hold very lightly, and move in another direction. You do not need to achieve complete balance in this first group of three because you still have the second group to work with.

Make your design dramatic. You might go from a reverse curve to a straight line because that is a good contrast. It is essential to decide how long to make the line. Then, move in the opposite direction and make the third curve—perhaps an accented curve—or another of your choice.

Solder this first design to your base, making it look like the wire rests on the wood.

Now, make your second group of three. Design the largest curve in your second group to complement the largest curve in the first group and position them with as much distance between them as possible.

Create a tensional relationship between the accents of the two largest curves. It will take a while to become sensitive to the tensions between curves. But persist. In time, you will instantly recognize the balance of all forces of movement and accents.

Combined with the first, the second group of three curves should balance directional forces from all directions. Keep turning the exercise around as you work to see it from all angles. Moreover, be sure to look at it from the top! Solder the ends of the first and second groups together only when you are satisfied with the design of the second group.

Remember, your objective in this problem is to find the most three-dimensional solution. Your lines should not delineate a volume. It is a common tendency, but it is inappropriate in this exercise. Your lines should look like they move separately in and out of space. Try to achieve lines that have the force of the curve. They have to move fast enough to support their positions. Avoid lines that look like they are made of wet string.

Beware of a “tweak” in which the end of one curve is the same as the end of the next (like a leaf). It may be decorative, but it is not nice here, and in this problem, there is nowhere to hide. All the curves are out there and exposed.

Try to stay spontaneous. You cannot approach this problem cold-bloodedly. The other problems may be approached more formally, but this one cannot be. You have to feel your way. It takes much emotional energy, so you might want to do one or two of these exercises every night instead of doing many all at once.

This problem is like approaching infinity. You never get there. However, the more you try, the more uses you will find for lines. This problem can be irritating because it looks easy but is not.

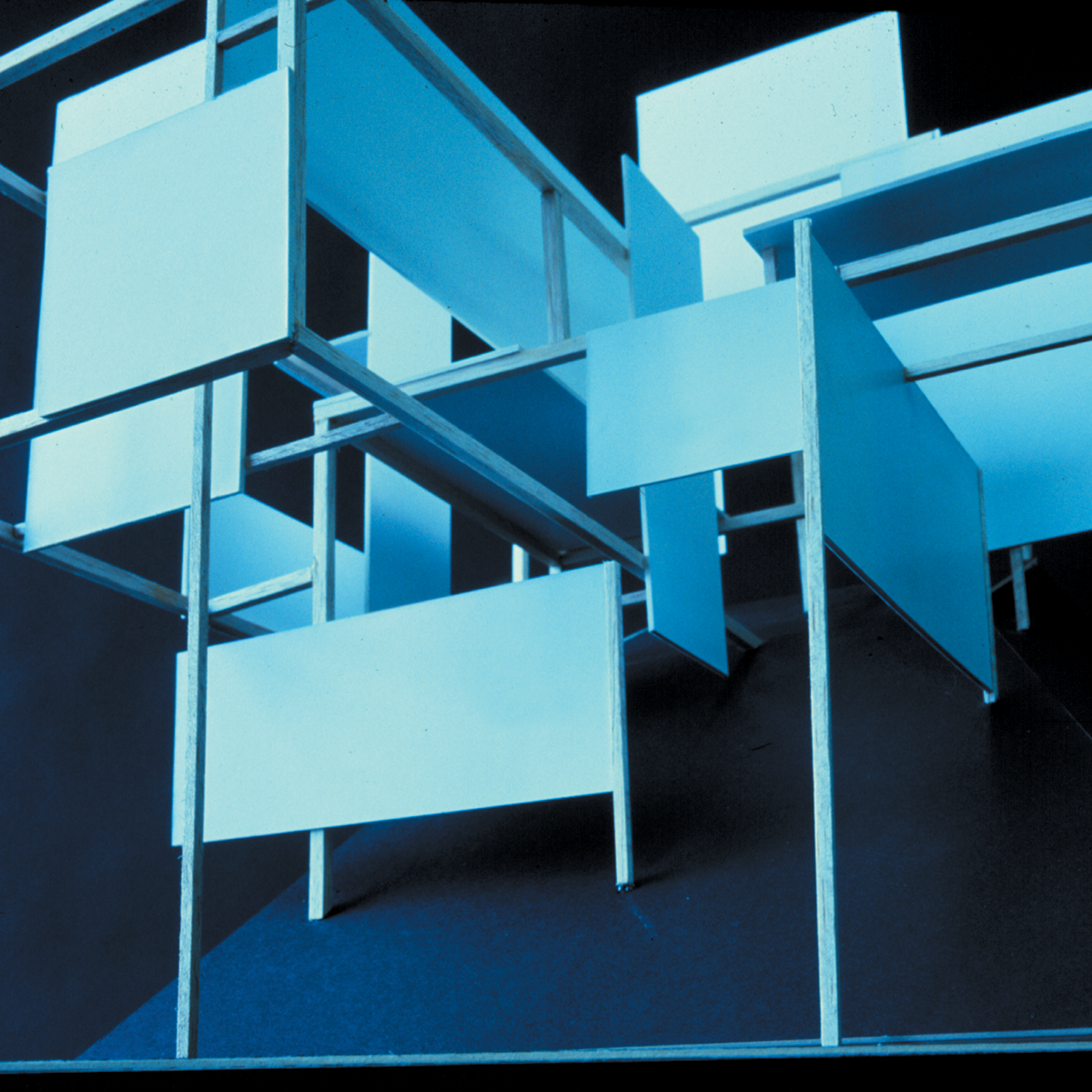
Understanding the wire problem can help you with any three-dimensional organization if appropriately used. However, it must be understood so well that you do not have to think much. It can then be used quickly, creatively, and emotionally to make proportion sketches, visualize the design of axes and grouping of axes, suggest the movement of planes, distort proportions meaningfully, and, in general, establish a very three-dimensional image.

Forward
The principles of study that Rowena helped formulate were embodied in a foundation curriculum that became the primary, universal first year of study for all Pratt Institute art and design students.
On Sketching
For those of you who have never worked in three dimensions, it provides an introduction to the three-dimensional world and to that complex and exciting set of relationships that will challenge you for the rest of your life.
Exercise One: Rectilinear
“At first working with 3-dimensional forms in this way is difficult. But soon you will begin to speak this language. You really have to make these beautiful. That sounds pretentious. How can you make three blocks beautiful? But I know that you can.”
Exercise Two: Curvilinear
“The second experience involves the organization of curvilinear volumes in a dynamic relationship. In addition to mass, proportion and character, you will now deal with the additional challenge of the diagonal axis. We will work with the following curvilinear solids.”
Exercise Three: Rectilinear + Curvilinear
“In this problem we introduce the notion of group movements. Be aware of inherent, comparative, and overall proportions.”
Exercise Four: Fragments
“The objective of this exercise is to choose a solid, cut it apart and reorganize the cut fragments in a new composition that is more beautiful than the original form from which it was derived.”
Exercise Five: Planar Construction
“The objective of this exercise is to gain an understanding of the characteristics of planes and how they relate to each other in space. In this problem you are asked to create a beautiful construction using a variety of planes.”
Exercise Seven: Construction
“The abstract experience based on construction involves the design and organization of contrasting forms, the new experience of grouping forms to create related movements, and a deeper understanding of the balance of directional forces and of tensional positions in space.”
Exercise Eight: Convexity
“The exercises in convexity and concavity are based on organic forms. They present the opportunity to explore the properties of a single, specific form. Unlike the dramatic quality of the construction exercise, the convexity and concavity exercises deal with subtle gesture.”
Exercise Nine: Concavity
“In the convexity exercise you were already learning about concavity — about how negative volume affects form. Now, the focus will be on concavity.”
Exercise Ten: Space Analysis
“The study of planes in space is all about relationships — about how the planes look in relation to each other. The answer is not yes or no — it’s yes in relation to something else or no in relation to something else.”
Exercise Eleven: Space Design
“This project follows the abstract exercises in space analysis and cannot be successfully completed until the student has mastered those exercises.”
Exercise Twelve: Development
“These are examples of more advanced work in which students continue to explore abstract forms but also begin to apply some practical criteria to their work.”